Euclid flourished about 50 years after Aristotle and was certainly familiar with Aristotle's Logic. Euclid's organisation of the work of earlier geometers was truly innovative. His results depended upon basic assumptions, called axioms and "common notions". There are in total 23 definitions, five axioms and five common notions in The Elements. The axioms are specific assumptions that may be considered as self-evident, for example "the whole is greater than the part".
Euclid imposed order on mathematics, creating an axiomatic system that endures to this day. His aim was to deduce a large number of theorems or propositions from a small number of axioms.
His approach may be summarised thus: (a) define your terms; (b) state your assumptions; (c) derive the logical consequences. With this approach, Euclid proved nearly 500 theorems from a handful of axioms. The deductions had to be logically unimpeachable, with each step following clearly from the one before.
But are Euclid's axioms really beyond question? Is it essential to assume that "the whole is greater than the part"? It this even justified? Galileo was among the first to cast doubt on it. He reasoned that the set of natural or counting numbers, 1, 2, 3, … and the set of even numbers, 2, 4, 6, … are of equal magnitude. It is a simple matter to make a perfect match between each number and its double: we pair 1 with 2, 2 with 4, 3 with 6, and so on. Since the two sets of numbers are infinite, the process continues forever.
Galileo concluded the quantity of even numbers is equal to the quantity of natural numbers. But this is counter-intuitive. The even numbers form only a part of the set of natural numbers. We can argue that, since the even and odd numbers can easily be paired, they are equally abundant. But, with Galileo’s logic, they are also equinumerous with the natural numbers. However, Galileo could not bring himself to deny Euclid’s axiom that the whole is greater than the part.
Great discovery
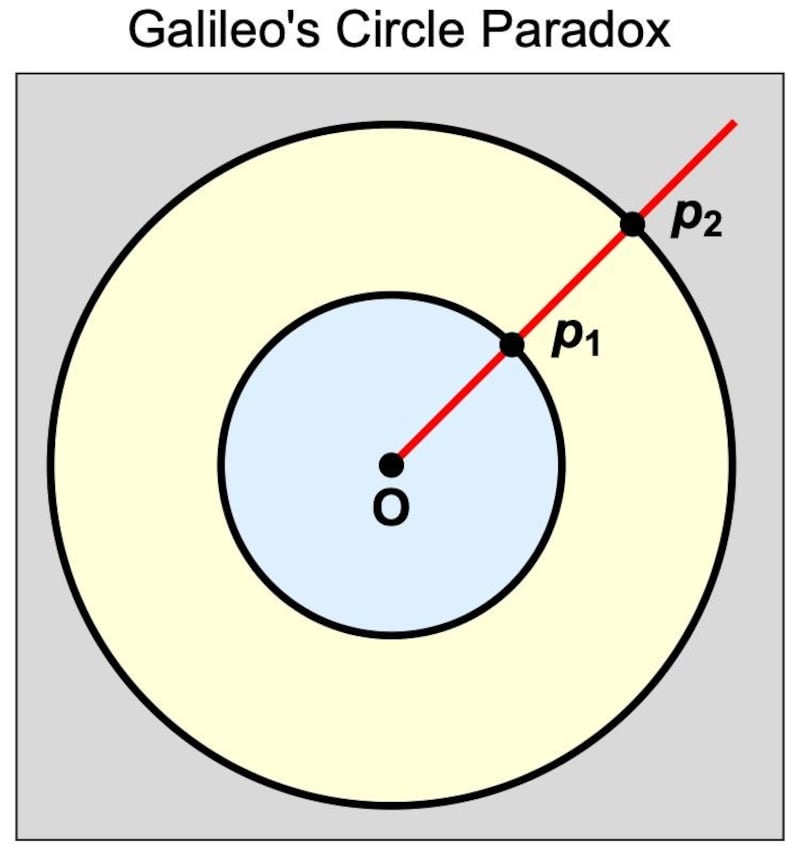
Galileo drew a similar conclusion about continuous sets. For two concentric circles, a radial line from the centre O cuts them both. Thus, every point p1 on the inner circle is matched to a point p2 on the outer one. Galileo concluded that there must be an equal number of points on each circle. But this is amazing: the outer circle is clearly longer than the inner one. Galileo concluded that "the attributes of equality, majority, and minority have no place in infinities". He was on the brink of a great discovery, but he did not develop his ideas. That had to await the arrival of Georg Cantor, the founder of modern set theory, about three centuries later.
Cantor recognised that the hallmark of an infinite set is that it can be matched one-to-one to a part of itself. He used this characteristic to define infinite sets. He then defined any two infinite sets that can be so paired to be of equal size. Thus, Cantor abandoned Euclid’s axiom and reasoned that, when it comes to infinite sets, “the whole is not necessarily greater than the part”.
So, we may ask, who was correct? Mathematicians are free to assume whatever system of axioms they find most useful or interesting. A system of axioms is consistent if it is impossible to deduce contradictory results from it. The choice depends on the purpose; for modelling the physical world, axioms that produce physically realistic results are required. For purely mathematical explorations, any consistent system can be used as a starting point. The search continues today for the most fruitful axiom systems.
A new collection, That's Maths III, has just been published by Logic Press, at https://logicpress.ie/
Peter Lynch is emeritus professor at UCD School of Mathematics & Statistics – he blogs at thatsmaths.com









